Note on Gibbs-Heaviside vector 'algebra'

P. Puska
Electromagnetics lab., Helsinki U. of Tech., Finland

Strictly speaking Gibbs-Heaviside (GH) vector algebra is not an algebra, but rather a combination of cross product algebra (an example of Lie algebra) and a scalar product. However, GH 'algebra' is contained in Clifford algebra ${\cal C}\ell_{3,0}$ generated by

$$\mathbf e_1^2=\mathbf e_2^2=\mathbf e_3^2=1,\qquad \mathbf e_i\mathbf e_j=-\mathbf e_j\mathbf e_i\,\,\,\,i \neq j.$$

In ${\cal C}\ell_{3,0}$ we can build a model of GH 'algebra' by making the identification

$$\mbox{scalar product of } {\cal C}\ell_{3,0} \leftrightarrow \mbox{scalar product of GH 'algebra'},$$

and using the identity relating the traditional cross-product and exterior product (denoted by wedge) of ${\cal C}\ell_{3,0}$:

$$\mathbf a \times\mathbf b = (\mathbf a \wedge \mathbf b) \mathbf e^{-1}_{123}, %$$

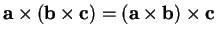whenever a,b are any real vectors in GH or ${\cal C}\ell_{3,0}$. Thus the non-associativity of the cross-product can be understood on the basis of the model of the cross-product we have just built in ${\cal C}\ell_{3,0}$. With the identity above it is straightforward to verify that $\mathbf a\times(\mathbf b\times\mathbf c)=(\mathbf a\times\mathbf b)\times\mathbf c$ does not hold in general.